The loophole-free quantum entanglement experiment (1): Bell’s Theorem.

Recently, a new experiment has attracted a lot of attention. It was published in Nature and entitled as “Loophole-free Bell inequality violation using electron spins separated by 1.3 kilometres” ¹. Many scientific and non-scientific media took account of the results of the experiment and published short news with fancy titles as Quantum ‘spookiness’ passes toughest test yet (Nature News), Quantum weirdness proved real in first loophole-free experiment (New Scientist) or Sorry, Einstein. Quantum Study Suggests ‘Spooky Action’ Is Real (The Times). It looks like something important has just happened, but it is difficult to grasp what it is. This is the purpose of this series of posts, to understand why this and others experiments are so important and the story behind them.

As always, this story has a beginning and, in this case, the beginning of the story has a popular main character, Albert Einstein. Einstein is known to be both a founder and a critic of quantum mechanics. His strongest complaint about this theory was summarized in this quote: “God does not play dice with the universe”. Almost everyone knows about this quote, but what does it really mean?

Quantum mechanics is a non-deterministic theory, meaning that it cannot predict the outcomes of experiments. It can only predict the probability of each possible outcome to each possible measurement perform in an experiment. To Einstein this proves that quantum theory was incomplete, meaning that it makes correct prediction, but it does not give all the possible information. He believed that there should be an underlying reality that is hidden to us and that quantum mechanics is only an approximation to this reality.

In 1935, Einstein published a paper with B Podolsky and N Rosen exposing what they thought it was a proof of the incompleteness of quantum mechanics ². In this paper, they combined two properties of quantum mechanics to construct an apparent contradiction between this theory and special relativity. The first property is the superposition of states, meaning that a quantum system can have more than one value for each parameter. This property is the origin of probabilities in quantum mechanics predictions because this superposition should collapse when this parameter is measured and then only one of the possible outcomes materializes in a random way. The second property used is the correlation between systems. Let see an example. If we have the simplest possible quantum system, a half spin particle, it has two possible outcomes for a certain measurement. We call these possible outcomes up and down. By the superposition of states, it is possible to create a particle that is both up and down. Thanks to correlations, we can create sets of particles that fulfill an “up and down rule”. For instance, we can create two particles with the condition that if one is up the second should be down. If we combine both properties we have a system with both particles in superposition but with one extra condition. If we separate the particles a very high distance and we look at one of them it will collapse into one of the two options. The important point of this thought experiment is that if we measure one particle and we find it up the other particle should immediately collapse to a down state. This happens immediately even if nothing interacts with the second particle and it is arbitrarily far away.

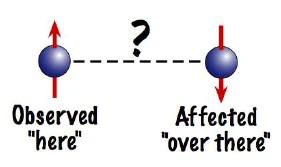
To Einstein, Podolsky and Rosen (EPR in short) their reasoning totally disproved quantum mechanics as a complete theory. To them, quantum mechanics predicted a faster-than-light way of communication in clear contradiction to relativity and it could not be correct. The scientific community was not convinced at all. The solution to this apparent paradox came from the original problem that bothered Einstein. As the outcomes of the measurements are random they cannot be used to transfer any kind of information, so the correlations between quantum systems do not contradict special relativity. This feature was called quantum entanglement and it slowly became the most important quantum feature. EPR paper was ignored until the 70s, becoming a canonical example of a sleeping beauty [note a].

If EPR paper was forgotten for almost thirty years why did it become so popular afterwards? To understand this, we have to jump to 1964 when a CERN scientist, John S. Bell, decided to analyze this problem. It is worthy to mention that Bell was not working full-time in studying the foundations of quantum mechanics. He was specialized in nuclear physics and studying quantum foundations was mainly a hobby. He was interested in the boundaries between quantum and classical mechanics, and in 1964 he published a seminal paper ³ where he formulated the Bell’s Theorem: “No physical theory of local hidden variables can ever reproduce all of the predictions of quantum mechanics”. This theorem mathematizes EPR claims, showing that if there is a hidden world where the predictions of quantum mechanics are not random then one of these two statements should be true: 1) Quantum mechanics predictions are incorrect, or 2) there are superluminal communications in clear contradiction to special relativity.

Until this moment, the debate was purely philosophical, but Bell gave it a twist. Together with his theorem, he calculated an inequality that could not be violated by local hidden variables theories but was violated by quantum mechanics. This transformed the philosophical debate into a scientific one. If we perform an experiment and we find a violation of this inequality (now called Bell’s inequality [note b]) we can conclude that the predictions of quantum mechanics are correct and, therefore, the universe is intrinsically random or there are faster-than-light signals.

It took more than fifteen years until someone performed a Bell-type experiment. 1981 Alain Aspect was a Ph.D. student and he decided to make a set of experiments showing Bell inequalities violations. During his Ph.D., Aspect published three papers ⁴, each one of them explaining a different experiment showing clearly the predictions of quantum mechanics. Unfortunately, neither of these experiments could be considered a definite experiment. This happens because the conditions impose by Bell’s Theorem are very difficult to achieve. For instance, you need the two systems to be spatially separated in order to perform measurements without any possible classical communication. You also need to have a good statistics, because Bell’s inequalities are based on mean values. Each of Aspect’s experiments contained a loophole, meaning that they failed in fulfilling, at least, one of the conditions (see ⁵ for a review on the different kind of loopholes). Closing all these loopholes has been proved to be an extraordinary task and it took more than thirty years to perform a loophole-free experiment. During these years the competition has been tough and many research groups have mainly focused in designing the perfect experiment. More interestingly, after thirty years of competition two different papers claiming to have solved the problem have been posted with only months of difference (Refs. [1] published in Nature and ⁶ still unpublished).

This series is about the race towards a loophole-free Bell’s experiment. We will discuss the different loopholes (locality, fair sampling, and free-choice) and the different attempts to overcome them. Finally, we will review the proposed loophole-free experiments and we will discuss their implications.

Notes:

[a] A paper that is not cited in a long time and becomes very popular at a certain moment is called a sleeping beauty.

[b] It is fair to mention that the original Bell’s inequality was not measurable in real experiments. During the following years, more realistic inequalities were proposed, being the most important the one proposed in: JF Clauser, MA Horne, A Shimony, and R.A. Holt. Proposed Experiment to Test Local Hidden-Variable Theories. Phys. Rev. Lett. 23, 880 (1969). This inequality is usually referred as the “CHSH Bell inequality”. In this and upcoming posts, we will use the term “Bell’s inequalities” for any inequality that is derived from Bell’s Theorem.