Quantum Thermodynamics II: The second law of thermodynamics

The second law of thermodynamics is one of the oldest and most tested physical laws. Unfortunately, it prevents us from doing very useful things, like creating a perpetual motion machine of the second kind. This law is extremely important for both theoretical and practical purposes and, because of that, it has been broadly studied since Clausius first formalized it in 1854. His formulation is now know as the Clausius statement:

“Heat can never pass from a colder to a warmer body without some other change, connected therewith, occurring at the same time”.

A different formulation comes from Lord Kelvin:

“It is impossible, by means of inanimate material agency, to derive mechanical effect from any portion of matter by cooling it below the temperature of the coldest of the surrounding objects”.

These two formulations can look different, but it is very easy to probe that a violation of one of them leads to a violation of the other one (see ¹ or any other book on basic thermodynamics).

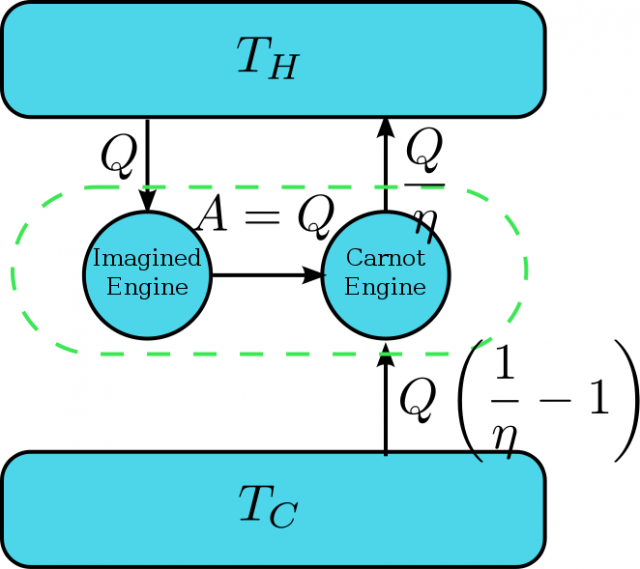
As usual in quantum thermodynamics, the relation between the second law of thermodynamics and quantum mechanics can be divided in two different problems. First, as quantum mechanics is the theory that describes the behavior of atoms and molecules the second law should be derivable from it. Second, it is not clear if a law stated from macroscopic principles is applicable to quantum systems at all.

The derivation of the second law from quantum mechanical principles has been attempted almost since quantum mechanics was born. In this direction, Von Neumann proved that if one basic principle of quantum mechanics, the uncertainty principle, is fully violated the second law can also be. The uncertainty principle states a limit to the knowledge that we can obtain for certain magnitudes of quantum systems. Von Neumann proved that if our knowledge about quantum systems is perfect we can create a machine that violates the second law ². This derivation assumes a full capacity to distinguish quantum states, but this condition can be relaxed. More recently³, Hänggi and Wehner showed that any theory that allows more information than that allowed by the uncertainty principle, even an infinitesimal improvement, violates the second law. Again, the demonstration is based on the construction of a machine that creates an impossible process by separating two states beyond what quantum mechanics allows.

Even if the second law can be related to the uncertainty principle the second question remains. Can it be applied to quantum systems? Recently, there have been some attempts to use quantum mechanical resources to improve the efficiency of thermal machines beyond classical constrains. One example is the use of quantum coherence, which is the property of quantum systems of being in a superposition of different states, as a resource. Scully et al. describe ⁴ a revolutionary method to extract energy from a single heat bath was presented. This method apparently violates Clausius statement, but in fact it does not because the device requires an external energy input in order to create the coherence. Once it is created, coherence acts as the fuel of the machine (it is sometimes called “phaesonium”) and it allows extracting heat from the reservoir more efficiently and faster than a classical engine.

Different methods for using quantum properties in thermodynamic machines have been proposed during the last years⁵⁶, but at the moment the second law is always preserved. On the other hand, a prove of its validity for quantum systems is lacking, and the possibility of creating a “quantum perpetual mobile of second kind” is still there.