Advances on an Erdős problem on convex boundaries
Among his around 1525 papers, Paul Erdős considered On sets of distances of n points 1 as his most important contribution to discrete geometry. There, he stated:
On the boundary of every convex body, there exists a point P such that every circle with center P intersects that boundary in at most 2 points.

(Note that throughout this post all the objects considered will be in the plane). This statement can be rephrased as:
On the boundary of every planar convex body, there exists a point P such that there are no more than 2 points equidistant to P on that boundary.
However, the conjecture turned out to be false. An easy counterexample is an acute triangle, for which any point on the boundary is the center of a circle intersecting that boundary in 4 points. The following figure shows this for a particular point, but you can check the points you want at this interactive GeoGebra construction.
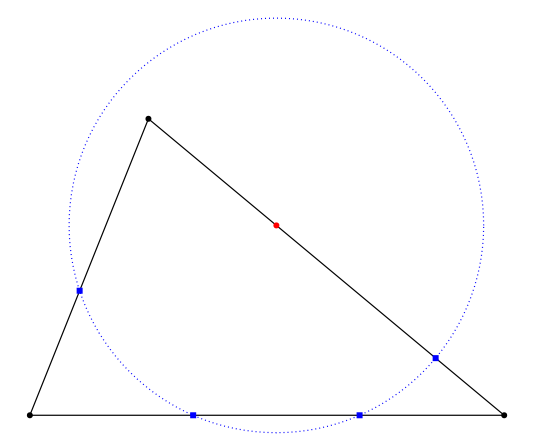
In fact, it turns out that any point on the boundary of a regular (2k+1)-gon is the center of a circle intersecting that boundary in 4 points. Naturally, a question arises:
Is this Erdős’s conjecture true when the upper bound 2 is replaced by 4?
Imre Bárány and Edgardo Roldán-Pensado give a negative answer to this question, constructing a convex body such that the smallest number for which Erdős’s statement holds is 6, i.e., such that for any point P on its boundary there is a circle with center P intersecting that boundary in 6 points.
The convex body they construct is a 15-gon. They consider the points ![]() ,
, ![]() ,
, ![]() , and
, and ![]() , together with the points
, together with the points ![]() and
and ![]() , for
, for ![]() , obtained by rotating the
, obtained by rotating the ![]() ‘s around the origin by an angle of
‘s around the origin by an angle of ![]() and of
and of ![]() , respectively. The 12-gon defined by these points looks like this
, respectively. The 12-gon defined by these points looks like this

and it can be checked at this interactive GeoGebra construction. The union of the shaded regions in the figure contains the polygonal chain ![]() and, using a technical lemma, this allows to prove that for any point
and, using a technical lemma, this allows to prove that for any point ![]() in some neighborhood
in some neighborhood ![]() of the polygonal chain
of the polygonal chain ![]() there is a circle with center
there is a circle with center ![]() that intersects the polygonal chain
that intersects the polygonal chain ![]() in at least 6 points.
in at least 6 points.
Another technical lemma, together with the fact of the angle ![]() being acute, implies the existence of a suitable point
being acute, implies the existence of a suitable point ![]() . By rotational symmetry, there also exist suitable points
. By rotational symmetry, there also exist suitable points ![]() and
and ![]() , such that
, such that ![]() for
for ![]() form the claimed 15-gon.
form the claimed 15-gon.
In view of this construction, Bárány and Roldán-Pensado 2 conjecture that
On the boundary of every convex body, there exists a point P such that every circle with center P intersects that boundary in at most 6 points (i.e., such that there are no more than 6 points equidistant to P on that boundary).
It is interesting to note that, both the original Erdős’s conjecture and this updated one, state the existence of a universal upper bound for the number of intersections (or equidistant points), independent of the convex body considered.
Although the existence of such a universal constant remains open, Bárány and Roldán-Pensado throw some light on the problem. They prove that for every convex body there is a finite upper bound as in the statement. More formally:
For every convex body
, there is an
being the smallest natural number for which there exists a point
on the boundary of
such that every circle with center
intersects the boundary of
in at most
points (i.e., such that there are no more than
points equidistant to
on that boundary).
This finiteness result is actually a consequence of a stronger one. For a fixed convex body ![]() , one can define the set
, one can define the set ![]() of pairs
of pairs ![]() in which
in which ![]() is a point on the boundary of the convex body and
is a point on the boundary of the convex body and ![]() is a normal to that boundary at
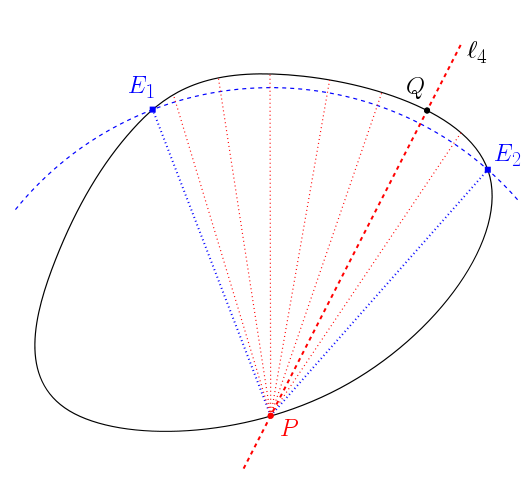
is a normal to that boundary at ![]() . See the following figure.
. See the following figure.

Note that there might be several pairs for which the normals intersect at a common point ![]() on the boundary of the convex body, like
on the boundary of the convex body, like ![]() and
and ![]() in the figure. What Bárány and Roldán-Pensado prove is that there exists a point
in the figure. What Bárány and Roldán-Pensado prove is that there exists a point ![]() for which this can only happen a finite number of times. More formally:
for which this can only happen a finite number of times. More formally:
Given a convex body
, there is a point
on the boundary of
for which there is a finite number of pairs
such that
and
.
The reason why this result implies the previous one deserves to be explained before finishing. Consider a point ![]() on the boundary of
on the boundary of ![]() such that there are 2 points equidistant to
such that there are 2 points equidistant to ![]() on that boundary, call them
on that boundary, call them ![]() and
and ![]() . The distance between
. The distance between ![]() and these two points on the boundary is the same.
and these two points on the boundary is the same.
Hence, because of Rolle’s theorem, there must be a point ![]() on the boundary, between
on the boundary, between ![]() and
and ![]() , which maximizes or minimizes the distance to
, which maximizes or minimizes the distance to ![]() . Then, for this
. Then, for this ![]() there is a normal
there is a normal ![]() which contains
which contains ![]() . See the following figure.
. See the following figure.
Hence, if ![]() is a point on the boundary of
is a point on the boundary of ![]() for which there are
for which there are ![]() pairs
pairs ![]() with
with ![]() and
and ![]() , then any circle centered at
, then any circle centered at ![]() intersects the boundary in at most
intersects the boundary in at most ![]() points, which implies that
points, which implies that ![]() . If the latter is finite, the former has to be finite as well.
. If the latter is finite, the former has to be finite as well.
As a final remark, note that this result does not imply the existence of a finite universal constant. There might exist a family of convex bodies ![]() with respective constants
with respective constants ![]() such that those
such that those ![]() tend to infinity. In that case, no constant
tend to infinity. In that case, no constant ![]() would be universal, since one could always find a convex
would be universal, since one could always find a convex ![]() for which
for which ![]() , i.e., for which that
, i.e., for which that ![]() would not be valid.
would not be valid.
In summary, this paper by Bárány and Roldán-Pensado includes two significant advances on an Erdős problem which, despite its simple formulation, remains unsolved since 1946.
References
- Paul Erdős. On sets of distances of n points. The American Mathematical Monthly 53(5) (1946), 248-250. http://www.jstor.org/stable/2305092 ↩
- Imre Bárány and Edgardo Roldán-Pensado. A Question from a Famous Paper of Erdős. Discrete and Computational Geometry. DOI: http://dx.doi.org/10.1007/s00454-013-9507-z ↩
2 comments
As far as I understand the conjecture says that there exists a point P, not that all the points have that special property. Therefore, I think that should not be stated that the conjecture is false because the example of the triangle.
Dear dramey. Here comes another explanation, which I hope you find helpful.
Let us focus on the (boundary of the) acute triangle. What Erdős’s statement says is that there exists a “special” point P on the triangle, where special means having the following property:
“Every circle with center P intersects the triangle in at most 2 points.”
Why is this is false? For any P you choose on the triangle, the Grinch can find a circle centered on your P and intersecting the triangle in 4 points.
Thus, the Grinch has showed that for your point P:
“Not every circle with center P intersects the triangle in at most 2 points.”
because there is one intersecting in 4 points. In other words, the Grinch has showed that your point P is not “special”.
But the Grinch can do the same for any point P you choose, so there is no “special” point and hence the statement is false.
Of course, the counterexample is not mine, I just try to explain it 🙂 You can play around and emulate the Grinch with the GeoGebra interactive construction for the triangle.