Quantum Thermodynamics I: Foundations and concepts

Both thermodynamics and quantum mechanics are very important theories, but they are applicable to very different kinds of systems. Thermodynamics describes macroscopic systems where the number of particles is huge (around Avogadro’s number: 6.022×10²³). On the other hand, quantum mechanics describes the behavior of microscopic particles. The regimes where these two theories can be applied are very different, but this does not mean that there are no connections between them. Some of these connections have arisen during the last years.

Mainly, there are two different ways of connecting quantum mechanics with thermodynamics. First, macroscopic systems are composed by many microscopic ones. Then it is clear that, if quantum mechanics is the correct theory to describe the behavior of atoms and molecules, thermodynamics should be an emerging theory from the underlying quantum reality. It should be possible to derive thermodynamics from quantum mechanics. In the next post of this series we will discuss some recent attempts to derive thermodynamics laws from first quantum principles ¹.

The second way of relating these two theories can be summarized in the next question: What happens if a quantum system interacts with a thermodynamic one? An example is a single atom interacting with a thermal bath, as the radiation that surrounds it. This question was ignored for a long time, as simple atoms were never controlled and measured. When quantum mechanics was developed it was considered just a theory of ensembles, and single quantum systems were only imaginary tools. Even Schrödinger stated ² (see also the discussion in ³):

. . . we never experiment with just one electron or atom or (small) molecule. In thought experiments we sometimes assume that we do; this invariably entails ridiculous consequences. [. . . .] In the first place it is fair to state that we are not experimenting with single particles, any more than we can raise Ichthyosauria in the zoo.

Luckily, modern techniques allow researchers to experiment with single atoms and particles. The Nobel Foundation recognized these techniques by giving 2012 Nobel Prize to S. Haroche and D.J. Wineland for “ground-breaking experimental methods that enable measuring and manipulation of individual quantum systems”. The development of these techniques leaded to new kind of problems, where single atoms and molecules can be analyzed when they are in contact with thermal baths. This connects the microscopic and macroscopic worlds. Furthermore, new theoretical approaches where developed in order to describe these experiments, as quantum jumps and master equations ⁴. Of course, different approaches present different features, but they are all equivalent as they are derived from the same principles. Most of the results we will discuss in these series of posts have been studied by the use of the master equation approach.

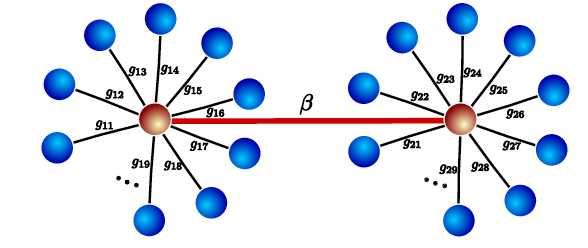
The master equation approach is based on modeling certain aspects of the dynamics of the system as stochastic processes. It has been used successfully in quantum optics and atomic physics for a long time ⁵. Only recently, the validity of some of the approaches used and their application to systems locally coupled to baths have been analyzed ⁶, with very interesting results. Master equations can be used in order to describe quantum systems, under certain assumptions as weak coupling to the baths, even if only part of the quantum system interacts with the bath (see figure 1). The method allows recent researchers to study interesting problems as energy transfer in quantum systems coupled to different baths, photosynthetic complexes or microscopic refrigerators. These applications and fundamental problems will also be discussed in following posts.

In summary, we will talk in the next posts about recent attempts of deriving and understanding thermodynamics from quantum mechanical principles and about the potential uses of quantum systems in contact with macroscopic ones. Some of the topics will be the derivation and understanding of the second law of thermodynamics, Fourier’s law of energy transfer and quantum refrigerators.