Our current image of atoms

By DIPC March 29, 2018 0 comments Print

The wave–particle dualism is a fundamental aspect of quantum mechanics. But, in order to explore this dualism properly and what it means for our image of atoms, it is necessary to review some ideas of probability.

image of atoms — Figure 1. Calculated 4f1 orbital of an electron’s eigenstate in the Coulomb-field of a hydrogen nucleus. Electrons in this state are 45% likely to be found within the solid body shown.

In some situations, no single event can be predicted with certainty. But it may still be possible to predict the statistical probabilities of certain events. For example, on a holiday weekend during which perhaps, say, 25 million cars are on the road in the European Union, statisticians, basing themselves on past experience, predict that about 400 people will be killed in car accidents. It is of course not known which cars in which of the Union countries will be involved in the accidents. But the average behaviour is still quite accurately predictable.

Along somewhat similar lines, physicists think about the behaviour of photons and material particles. There are basic limitations, like the uncertainty principle, on the ability to describe the behaviour of an individual particle. But the laws of physics often make it possible to describe the behaviour of large collections of particles with good accuracy. Schrödinger’s equation for the waves associated with quantum particles gives the probabilities for finding the particles at a given place and a given time; it does not give the precise behaviour of an individual particle.

In order to see how quantum probability works, consider the situation of a star being photographed through a telescope. In the case of waves striking a barrier in which there is a single opening about the size of the wavelength, the image formed on a screen behind the opening is not a precise point. Rather, it is a diffraction pattern, a central spot with a series of progressively fainter circular rings. The image of a star on the photographic film in a telescope would be a similar pattern.

Imagine now that you wish to photograph a very faint star. If the energy in light rays were not quantized, it would spread continuously over ever-expanding wave fronts. Thus, you would expect the image of a very faint star to be exactly the same as that of a much brighter star, except that the intensity of light would be less over the whole pattern. However, the energy of light is quantized; it exists in separate quanta, “photons,” of a definite energy, which obey Schrödinger’s equation. A photon striking a photographic emulsion produces a chemical change in the film at a single location, not all over the image area. That location, however, is not predictable in advance. All that we can predict is the probability that the photon might arrive at that location.

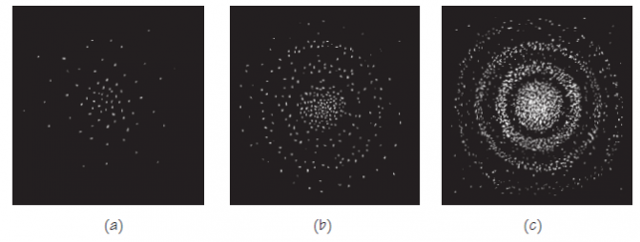
If the star is very remote, only a few photons per second may arrive at the film. The effect on the film after a very short period of exposure would be something like the pattern in Figure 3 (a). As the exposure continues, the effect on the film would begin to look like (b). Finally, after many photons have arrived from the star, a pattern like (c) would be produced, just like the image produced by a much brighter star with a much shorter exposure.

For huge numbers of particle-like photons, the overall distribution they form is very well described by the distribution expected on the basis of the wave intensity of light. For small numbers of quanta, the wave intensity is not very useful for predicting where the photons will go. One might expect them to go mostly to the “high-intensity” parts of the image, but one cannot predict exactly where for an individual photon. These facts fit together beautifully if you consider the wave intensity at a location to indicate the probability of a photon going there!

A similar connection can be made for de Broglie waves and particles of matter. For this purpose, rather than considering a diffraction pattern formed by an electron beam, consider an electron wave that is confined to a particular region in space. An example is the de Broglie wave associated with the electron in a hydrogen atom, which is spread out all over the atom.

Another example is the de Broglie wave of an electron in a good conductor of electricity. The wave’s amplitude at some location represents the probability of the electron being there, if a measurement of the electron’s location were to be performed.

According to quantum mechanics, then, the hydrogen atom does not consist of a localized negative particle (an electron) moving around a nucleus as in the Bohr model. Indeed, the theory does not provide any fixed, easily visualizable picture of the hydrogen atom. A description of the probability distribution is the closest thing to a picture that quantum mechanics provides (Figure 1).