Read it twice: heat transfer from a cooler body to a hotter body

Without any conflict with the second law of thermodynamics, heat can flow from a cooler but constantly heated body to another thermally connected and constantly hotter body. This anomalous heat transfer has been demonstrated in a two-phase liquid-vapor system composed of a Rayleigh–Bénard convection (RBC) cell filled one-half with normal liquid helium and one-half with helium vapor. Pavel Urban (Institute of Scientific Instruments, Academy of Sciences of the Czech Republic, Brno, Czech Republic) and his colleagues report in the PNAS journal ¹ that the observed anomalous heat transfer in the RBC cell occur because of evaporation and condensation processes in this closed cryogenic system which is maintained very far from equilibrium. This latter requirement is essential to prevent the violation of the second law of thermodynamics ².

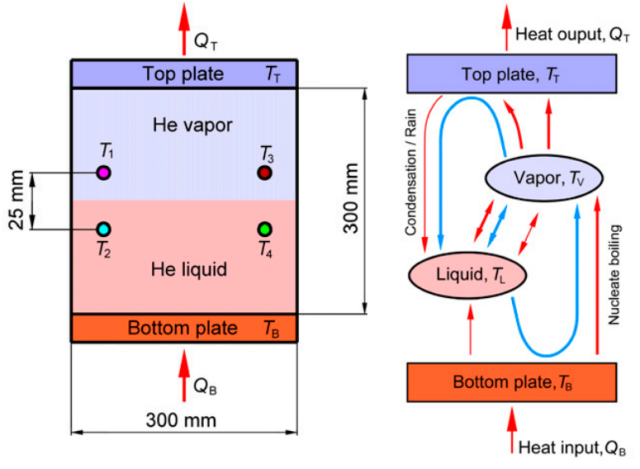
A Rayleigh–Bénard convection cell is a recipient with a fluid between two metallic plates and a heater below the bottom plate. Urban et al. [1] used a cylinder with 30 cm in both diameter and height, filled with liquid helium, whose boiling point is 4.2 K, and helium gas, whose vapor-liquid critical point is 5.195 K (let’s recall that above this temperature the distinction between liquid and gas phases do not exist). The thermal and fluid flows in RBC cells are characterized by the non-dimensional Rayleigh (Ra) number, the ratio for the rate at which potential energy is liberated because of buoyancy to the rate at which it is dissipated through viscous and thermal diffusion. The RBC cells with helium are capable of running at the largest Ra values attainable to date in the laboratory under controlled conditions, in fact, up to 10¹⁵. The copper bottom plate is heated with a resistive heater, but the temperature of the copper top plate is not controlled. Four small germanium thermometers continuously monitor the temperatures inside the RBC cell.

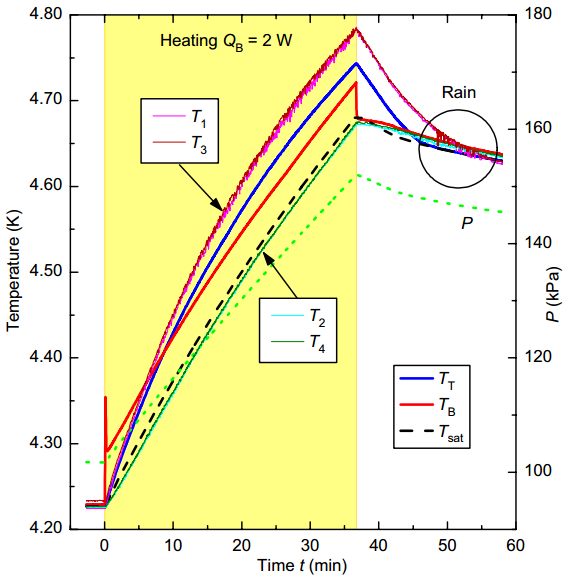
For an isolated RBC cell consisting of liquid and vapor in equilibrium, one would expect that slow heating of the bottom plate would result in partial evaporation of the liquid and uniformly rising temperature of the entire system. However, that this is not the case in the experiments of Urban et al. [1], as shown in Figure 2. After turning on the heater at t=0, the bottom plate temperature (T_B) peaked quickly with respect to the temperature of the liquid, resulting in boiling on the surface of the plate. Here on, all the rest of temperatures grow up gradually. The liquid temperatures (T₂ and T₄) follow the equilibrium saturation temperature (T_sat), but vapor temperatures (T₁ and T₃) and that of the top plate (T_T) rise faster than T_B, remaining above T₂ and T₄. When T₁ and T₃ cross T_B the vapor becomes the hottest subsystem and, soon, even the cooled top plate becomes hotter than the heated bottom one, resulting in the inversion of the heat flow.

The second law of Rudolf Clausius (1850) states that “it is impossible for any system to operate in such a way that the sole result would be an energy transfer by heat from a cooler to a hotter body” ³. However, heat can transfer from a cooler body to a hotter body if other effects occur within the system, like in air conditioners and refrigerators, but power input is required. The paradoxical behaviour of the RBC cell, with heat transferred from the cooler bottom plate to the hotter top plate, do not contradict the second law of thermodynamics even without the need for external work. The key to understand this phenomenon is the complex non-equilibrium and unsteady processes inside de RCB cell at extremely large values of the Ra number.

The heat supplied at the bottom plate partly passes the RBC cell and comes out at the top one, and partly is absorbed by the liquid layer above it and carried to the vapor phase directly, increasing its density and pressure. The latter is accomplished by means of vapor bubbles created at surface defects of the bottom plate, and by condensation on the upper plate and rain formation. In this sense, the RBC cell is reminiscent of a weather system, similar to the Earth’s water cycle, although this system consists of helium only, unlike water vapor being one of the many constituents of air.

To further demonstrate the anomalous heat transfer, the experiment of Urban et al. [1] ends with an inversion of the plate’s temperatures, with T_B>T₁≅T₂≅T₃≅T₄>T_T≅T_sat (see the encircled curves for later times in Figure 2). In such a region, heat is flowing from a colder body (the bottom heated plate) to a now hotter one (the cooled upper plate), which formally corresponds to negative Rayleigh numbers. In this period droplet condensation at the top plate of the RBC cell enhances heat transport by two-phase turbulent thermal convection. During the “rain” in the cell, liquid helium droplets are formed at the top of the RBC cell, but they either do not reach the bottom plate or evaporate upon reaching it.

In order to understand the physics of the RBC cell, Urban et al. [1] have developed a phenomenological model that describes the process quantitatively and shows that the second law of thermodynamics remains unchallenged. The observed anomalous heat transfer and the rain formation in the RBC cell occur because of the changes of phase, specifically boiling and condensation, which occur far from equilibrium.

In my opinion, it is very surprising that a small change in the RBC experiments, to partially fill the RBC cell with liquid, leaving the rest occupied by the saturated vapor, has resulted in such an unexpected behavior like anomalous heat transfer. Perhaps the authors of Ref. [1] expected that the use of a two-phase fluid instead of a single-phase one radically changed the dominant processes from boundary-layer growth and bursting to recondensation and evaporation at the liquid-vapor interface. However, the observation of a complete “weather system,” with evaporation, conduction, convection, and condensation, in a pure fluid system had to be a big surprise even themselves.

Some readers may ask themselves if the “weather system” that develops within the RBC cell can be considered as a thermal analogue to a perpetuum mobile. There is nothing to worry about, this process cannot be continued indefinitely and eventually the liquid layer is either evaporated or the average temperature exceeds the critical temperature, 5.195 K. In either case, a single-phase (gaseous) RBC cell remains without anomalous heat transfer.