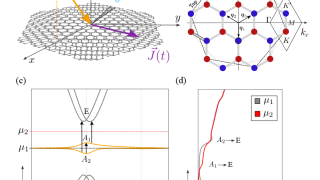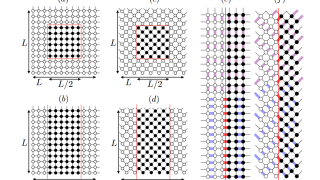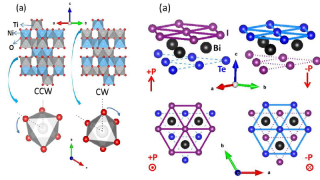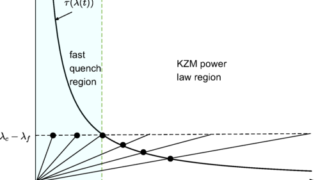
Topological water waves
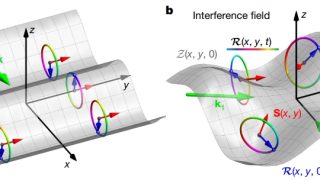
In physics, waves often reveal more than meets the eye. A ripple on a pond looks simple enough, but beneath its surface lies a rich mathematical structure that can connect something as everyday as water to the abstract worlds of optics, acoustics, and even quantum mechanics. A recent work brings this connection vividly to life […]