The vectorial features of the boundary-bulk correspondence in 3D Chern insulators
Some materials have special universal properties protected against perturbations. Such properties are theoretically described by topology, a branch of mathematics concerned with the properties of geometrical objects that are unchanged by continuous deformations. So-called topological insulators are electronic materials that have a bulk band gap like an ordinary insulator but have conducting states on their boundaries, i.e., edges or surfaces. The conducting surface is not what makes topological insulators unique, but the fact that it is protected due to the combination of spin-orbit interactions and time-reversal symmetry.
A topological invariant is a geometrical quantity that remains unchanged by continuous deformations. Topological invariants have found widespread applications in physics, chemistry, and materials science. One of the best known topological invariants in condensed matter physics is the Chern number.
The definition of the Chern number is not exactly simple. But it could be enough to understand the Chern number as an integer that characterizes the topology of filled bands in two-dimensional lattice systems. A band with a non-zero Chern number is topologically non-trivial. When the highest occupied band is non-trivial and completely filled, the state is called a topological insulator. A material whose topological phases can be characterized by the Chern number is called a Chern insulator, a class of topological insulators.
But when we make Chern insulators interact with light, something interesting happens. Electrons are spin-1/2 particles, whereas photons are spin-1 particles. The distinct spin difference between these two kinds of particles means that their corresponding symmetry is fundamentally different. An electronic topological insulator is protected by the electron’s spin-1/2 (fermionic) time-reversal symmetry; however, due to photon’s spin-1 (bosonic) time-reversal symmetry, the same protection does not exist under normal circumstances for a photonic topological insulator. In other words, we could have a Chern photonic insulator with broken time-reversal symmetry. Time reversal symmetry broken topological phases provide gapless surface states protected by topology, regardless of additional internal symmetries, spin or valley degrees of freedom. Thus, the topology of the propagation of light in photonic crystals has been the subject of much recent attention.
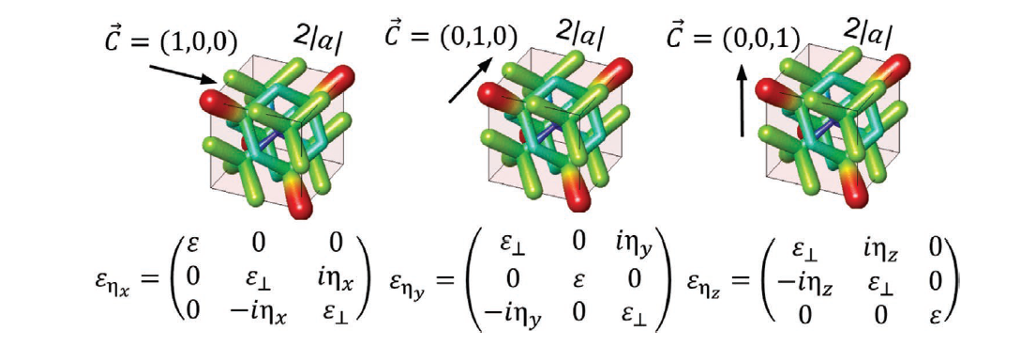
Formally, a 3D Chern insulator is a time-reversal symmetry broken topological phase characterized by a Chern vector C =(Cx ,Cy ,Cz ) that can support anomalous surface states on surfaces parallel to the Chern vector. In 2D, the Chern vector is always orthogonal to the plane of the system and, consequently, it can be regarded as a scalar quantity: the Chern number we mentioned before. In contrast with 2D or layered materials, where the vectorial nature of the boundary-bulk correspondence does not show up, the possibility of orienting Chern vectors in space demonstrated in photonic crystals open up the possibility of constructing domain walls between different orientations, and thus a definition of a proper vectorial boundary-bulk correspondence is required.
Do individual components of the Chern vector contribute in a linear independent way to surface modes? How do multiple photonic surface modes hybridize with each other with respect to the different orientations of the Chern vector? Is there an easy way of counting surface modes or predicting their direction? Finding answers to these questions is a challenging theory exercise, lacking of a 2D analogy. In search of those answers, a team of researchers explored 1 the possible interfaces between 3D photonic Chern insulators, focusing on possible changes in Chern vector orientation.
The researchers found that, for a 3D Chern insulator crystal, the Chern vectors across the interface no longer need to be parallel or anti-parallel to each other, which may render the scalar analogy with 2D difficult to apply. They demonstrate that vectorial features of the boundary-bulk correspondence need to be taken into account to correctly predict the number and the propagation direction of topological photonic surface modes, thus completing the vectorial boundary-bulk correspondence picture for 3D Chern insulator photonic crystals.
Beyond theoretical concerns, constructing interfaces with Chern vectors of different orientations is relevant for practical applications. Photonic 3D Chern insulator interfaces are a potential platform for unidirectional optical channels protected from backscattering. Furthermore, photonic crystal architectures with multiple Chern vectors of different orientation could enable new ways to control light propagation.
More on the subject:
3D topological photonic crystals whith Chern vectors at will
Author: César Tomé López is a science writer and the editor of Mapping Ignorance
Disclaimer: Parts of this article may have been copied verbatim or almost verbatim from the referenced research papers.
References
- Chiara Devescovi, Mikel García-Díez, Barry Bradlyn, Juan L. Mañes, Maia G. Vergniory, Aitzol García-Etxarri (2022) Vectorial Bulk-Boundary Correspondence for 3D Photonic Chern Insulators Adv. Optical Mater. doi: 10.1002/adom.202200475 ↩